Here, we received a claim that a solution was found again. It should not be terribly hard. Enjoy...
Key words: Geometry, Unusual geometry, Math, Physics, Chemistry, High school, Geometry Olympiads, Free Geometry, Euclidean Geometry, Calculus, Geometric Construction. Oh yes, going-nowhere discussions, haikus, and poems too.
Tuesday, July 19, 2011
Fox 9
Here, we received a claim that a solution was found again. It should not be terribly hard. Enjoy...
Thursday, July 14, 2011
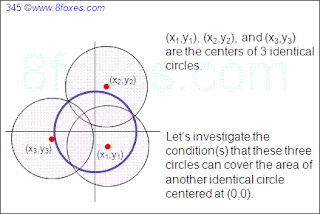
Fox 345
Sunday, June 26, 2011
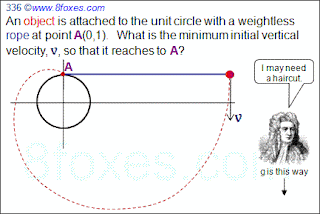
Fox 344
 http://www.8foxes.com/
http://www.8foxes.com/Two flow together
one rises one falls
one after another
one shines one glooms
Wednesday, June 22, 2011
Fox 343

What makes a man a man
is not the fire
nor the bullets,
but pure,
simple,
childish forgiveness.
-- Dervish Fox
Tuesday, June 14, 2011
Saturday, June 4, 2011
Fox 339 - Solutions
Thursday, May 19, 2011
Friday, May 6, 2011
Friday, April 29, 2011
Fox 330 - Solutions
Saturday, April 16, 2011
Fox 339
 http://www.8foxes.com/
http://www.8foxes.com/Monday, April 11, 2011
Fox 338

Thursday, April 7, 2011
Fox 337
Saturday, March 26, 2011
Fox 336
Thursday, March 24, 2011
Fox 335
Monday, March 21, 2011
Fox 334
 This is more like a google search than a geometry problem, but it is nice to have a reference in our blog as well. Good luck to those who want to try!
This is more like a google search than a geometry problem, but it is nice to have a reference in our blog as well. Good luck to those who want to try!Monday, March 14, 2011
Fox 333

Red Fox: Happy Pi+1 Day!
Himalayan Fox: What is that exactly?
Red: Well, March 14 was the Pi Day, you know, 3.14, and today is March 15th.
Himalayan: But Pi+1 would be 4.14 which is April 14th, isn't it?
Red: Oh, I didn't think that way. Whatever it is, happy March 15th to you brother.
Himalayan: OK, I'll mechanically say "to you too", but can we really happy while thousands of souls swept away with the water?
Red: Well, we can not die with the dead.
Himalayan: But we can help the living.
Red: I hear you brother.
Himalayan: Then we can celebrate 2Pi Day a few months later.
Wednesday, March 9, 2011
Balanced Mancala Problem

We have stones coming in batches. Each stone has a color and a weight. If the color of a stone is:
Yellow: It must be placed every other pit (every 2 pits) Batch size is always: 6 Total batches: y Total number = 6y
Red: Must be placed every 3 pits Batch size: 4 Total batches: r Total number = 4r
Green: Must be placed every 4 pits Batch size: 3 Total batches: g Total numbers = 3g
Blue: Must be placed every 6 pits Batch size: 2 Total batches: b Total number = 2b
Purple: Must be placed only once in 12 pits Batch size: 1 Total batches: p Total numbers = p
So there are N=6y+4r+3g+2b+p many stones. The stones in the same batch have the same weight. Different batches may have different weights. WLOG, assume that all weights are integers. We have a proof that ending up with the best well-balanced mancala is very difficult (NP-Hard). Here "well-balanced" means that the pit with the maximum weight is minimized when all stones are distributed. Let's call this maximum pit weight as W.
Consider the following heuristic process:
Step 1. Sort the batches with respect to their weights (batches with the high-weight stones go first) Step 2. Insert the first batch starting from pit number 1. Step 3. Insert the next batch in a way that the total maximum weight throughout 12 pits remains minimum. Step 4. Repeat Step 3 until all batches are placed in the mancala. Let H be the maximum weight throughout 12 pits.
A simple Example: Suppose we have only 4 batches: Yellow (6 stones, each 45 grams) Blue (2 stones, each 40 grams) Yellow (6 stones, each 30 grams) Green (3 stones, each 20 grams) First batch (Yellow) goes to pits: 1, 3, 5, 7, 9, and 11. H=45. Second batch (Blue) goes to pits: 2 and 8. H=45. Third batch (Yellow) goes to pits: 2, 4, 6, 8, 10, and 12. H=70. Fourth batch (Green) goes to pits: 3, 7, and 11. H=70.
In this exercise, heuristic actually finds the optimum, i.e., H=W=70 grams, observed in pits 2 and 8.
And the question: Prove that the worst-case of the heuristic solution, H, is always less than 2W. In other words, W ≤ H ≤ 2W always holds. If you disagree, then try to generate a counter-example.
Good luck!
Monday, March 7, 2011
Fox 332
Monday, February 28, 2011
Wednesday, February 23, 2011
Sunday, February 20, 2011
Fox 329
 See a similar concept: Bertrand Paradox
See a similar concept: Bertrand ParadoxDervish Fox: How can you cut a square randomly?
Red Fox: Here is a way; first generate a random number to represent point A. Then another random number for point B along the three others sides. Drawn the line AB, and cut it out.
Dervish: And how can you generate a random number?
Red: Excel does it easily. Type "=RAND()", and boom, you get one right away.
Dervish: But that depends on some seed numbers in the machine's memory, no?
Red: Yes, that's why they call them pseudo-random numbers. Do you have a problem with that?
Dervish: I have a bigger problem than that.
Red: So... you believe that random numbers can't exist.
Dervish: I believe that the word "random" should be removed from all world dictionaries.
Red: Radical.
Thursday, February 17, 2011
Fox 325, 326, 328 - Solutions
Fox 325 by Bob Ryden:

Fox 325 by six:
Fox 326 by Bob Ryden:
Fox 326 by Binary:
Fox 328 by César Lozada:
Fox 328 by Bob Ryden:
Saturday, February 12, 2011
Thursday, February 10, 2011
Fox 15 - Solution
Chrome, Firefox, Opera, Safari, or IE Explorer 9.0.
Tuesday, February 1, 2011
Fox 327
Earth is not a perfect sphere,
It's a geoid of some kind,
Not perfectly-round but close enough.
A year is a little more than 365 days.
A day is not exactly 24 hours,
little short of that.
Pi is more than 3.
Twenty-two over seven is not far off though,
often close enough.
Man hardly lives a 100 years.
Few goes beyond,
Some get close enough.
Truth ascends above digits,
distorts formulas,
bends orbits.
Man searches it with greed,
never reaches,
never conquers.
So my dear,
give up the precision,
stop running the numbers.
Instead,
smell the soil after rain,
hold a cuddling baby in your hand,
wander your vision from Vega to Orion,
let a snowflake die in your palm.
hear what morning breeze tells,
Then round up what you got.
A little more or a little less.
Even if it is still not found,
You're close enough.
--- Polar Fox
Tuesday, January 25, 2011
Sunday, January 23, 2011
Fox 325
Sunday, January 16, 2011
Fox 320 - Solutions
 This fox has been discussed extensively. See here...
This fox has been discussed extensively. See here...1. Calculus by Bleaug
He says: "I spotted this problem in a Paul Halmos book "Problems for mathematicians, young and old, 1991", actually in a French translation. He provided the solution below (reformulated by myself) as being proposed by Hugh Montgomery in 1985 in some Math Conference."

2. Checkerboard Solution by Rochberg and Stein:
- Call sub rectangles as TILEs.
- Start from the lower left corner of the overall rectangle (let's call this point as the origin)Draw horizontal and vertical lines separated by 1/2=0.5 units starting from the origin.
- This will create 0.5 x 0.5 squares (and possibly rectangles around 2 edges -top and/or RHS)
- Color the first square (that has the origin as one of its corners) as black the next one as white, and so on to generate a checkerboard-look.
- Each TILE will have equal areas of black and white (Why?)
- Therefore the overall rectangle will have equal areas of black and white.
- So overall rectangle has at least one integer side.
H means the horizontal side is integer, and V means that the vertical side is integer. a1 is the square at the origin. There could have been a smarter combination, but this simple one illustrates the process clearly. This looks like the closest geometric solution we can get -at this time.
 When there's hardly no day nor hardly no night
When there's hardly no day nor hardly no nightThere's things half in shadow and halfway in light
3. Induction by Robinson
- Assume that each H-tile has a width of 1, and each V-tile has a height of 1. Note that any rectangle can be converted this way without distorting the original problem. (This may increase the number of tiles significantly though)
- Chose any H-tile, say T(0). (If there is no H-tile, then the result is immediate)
- If there are H-tiles whose lower border shares a segment with T(0)'s upper border, choose one and call it T(1).
- Otherwise only V-tiles share this border. In this case, we can expand T(0) upward 1 unit. This does not increase the number of H-tiles. Also, the cut V-tiles still have height 1. (They are still V-tiles)
- Continue expanding T(0) until either the top of the rectangle is reached or a choice of an adjacent H-tile T(1) is possible.
- Then repeat the same process from T(1). (Continue upward similarly from T(1) to get T(2), and so on...)
- This will result in a chain of T(0), T(1), T(2), ... , T(m).
- Starting from T(0) again, work downward similarly to obtain a bigger chain:
T(-n), T(1-n), ... , T(0), T(1), ... , T(m-1), T(m) of H-tiles stretching from bottom to top. - Remove these tiles and slide the rest together to get a rectangle with fewer H-tiles.
- Induction applied to this smaller rectangle yields the result for the original rectangle.
Sunday, January 9, 2011
Fox 15

Friday, January 7, 2011
Fox 319 - Solutions

Trigonometry and calculus by Six:
x + y + z = 360 and sinx*siny*sinz
-> f(x,y) = sin(x)*sin(y)*sin(360-x-y)
-> f(x,y) = sin(x)*sin(y)*-sin(x+y)
Now to find the critical points of f(x,y), we just need to find the partial derivative with respect to x and y and solve for 0.
f_x(x,y) =
sin(x)sin(y)(-cos(x+y)-cos(x)sin(y)sin(x+y)
solve for 0.
sin(x)sin(y)(-cos(x+y)-cos(x)sin(y)sin(x+y)=0
-> tan(x) = -tan(x+y)
Since the function is symmetric, we should get the same partial derivative for y.
-> tan(y) = -tan(y+x)
-> tan(x)=tan(y)
-> x = y or they are opposites. However, if they are opposites, the original function just becomes 0. Thus, x = y.
Now substitute in x for y in the original equation and find its critical points.
Eventually, you will get sin(3x)=0
x = 120 degrees
y = 120 degrees
z = 120 degrees
Answer (D)
Monday, January 3, 2011
Fox 324
Saturday, January 1, 2011
Happy new year everybody!
 A new year,
A new year,a new beginning,
An unheard adventure - even it makes you late for dinner,
a new beginning.
Cold air that you breath in,
A warm shoulder that you can lean
A promise you've given to yourself - one more time,
a new beginning.
A morning sky painted in the East,
A colorful sunset as it fills the West,
Bread, cheese, water - and the rest,
a new beginning.