We heard a call from South,
saying, "Come - whoever you are!"
"Poor or rich"
"Full or null"
"Up or down"
"Live or dull"
"Come, just hear my cry!"
It was not the ears heard the call,
But the heart trembled with it.
How can one remain unconscious to this call?
So my friend,
never reject a good call.
Shine with your mercy
upon everyone.
Break your daily routine,
Do things out of the box.
Search the good in bad,
And never give up thinking.
--- Polar Fox






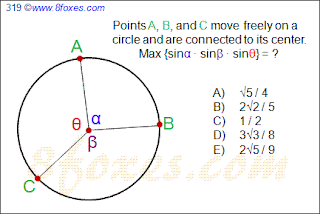


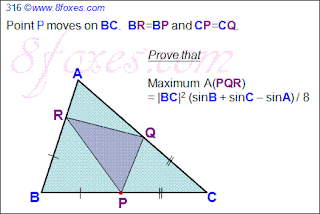































 The solution commented out by Julian deserves to be presented here. We often had questions inspired from the "things" we observe around us. Julian's solution is the other way. It uses a very casual observation from real-life for the solution. That's perspective lines converge linearly. See the solution below:
The solution commented out by Julian deserves to be presented here. We often had questions inspired from the "things" we observe around us. Julian's solution is the other way. It uses a very casual observation from real-life for the solution. That's perspective lines converge linearly. See the solution below: by Julian: This one has a simple, intuitive proof. Imagine a long, hollow square-prism. Something like a cardboard tube with a square cross-section. Imagine lines connecting the diagonally-opposite corners of the opposite ends. Due to the symmetry of the tube these four lines will meet at a single point half-way along it.
by Julian: This one has a simple, intuitive proof. Imagine a long, hollow square-prism. Something like a cardboard tube with a square cross-section. Imagine lines connecting the diagonally-opposite corners of the opposite ends. Due to the symmetry of the tube these four lines will meet at a single point half-way along it.






